Mapping Hammock Valley in the Forest Primes (K/1)

I recently came across an article outlining the difference between academic and intellectual goals in the early years. The article reminds us that,

“Academic goals are those concerned with acquiring small discrete bits of information, usually related to preliteracy skills and practiced in drill, worksheets, and other kinds of exercises designed to prepare the children for later literacy and numeracy learning. These require correct answers and rely heavily on memorization, not understanding…Intellectual goals and their related activities, on the other hand, address the life of the mind in its fullest sense, including a range of aesthetic sensitivities. The concept of intellectual emphasizes reasoning, hypothesizing, predicting, analyzing, imagining, and the development of ideas and the quest for understanding." (Chard, 2016)

In the creation of curriculum for the Forest Primes, we aim to encourage and motivate children to seek mastery of academic skills, in service of their intellectual pursuits. Our ongoing investigation of maps is an example of this in action. As you look through our documentation, I invite you to notice the excitement, motivation, and joy that’s abuzz as children use mathematical thinking and early literacy skills to engage with their work.

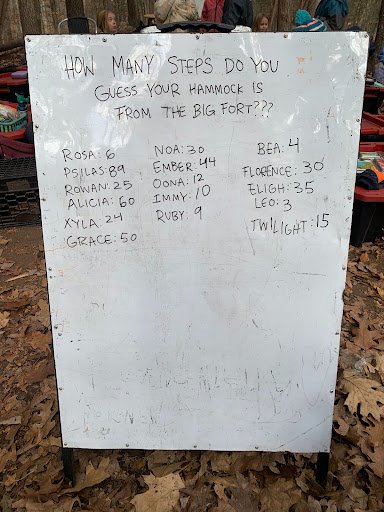
The area of our basecamp called “Hammock Valley” is currently covered with leaf litter, and a popular choice during morning play is the creation of paths through the leaves. Each day a network of pathways is constructed, and the following morning wind and new leaves have disappeared the paths away. Early in our week, children were challenged with estimating how many steps it might take to reach their hammock from The Big Fort at the center of Hammock Valley. 

Every child signed up for time throughout the week to determine the actual number of footsteps from The Big Fort to their hammock. During each of their turns, friends counted their footsteps, and then added their information to a collaborative map of Hammock Valley.

With every new path drawn came new opportunities to compare the different distances. Throughout morning play, children continuously stopped by to see what had changed.

Eligh: Florence has more than you Rosa…75. 

Val: Oh yeah! Looks like Rosa was 60 steps.

Rosa: Florence has more than me.

Eligh: Yep! 75 is more than 60. 

Rosa: Who else has more than me? Everyone?

Eligh: Not everyone!

Val: I wonder if there’s anyone who has a number really close to Rosa. Whose hammock is about as far away from the big fort as yours? 

Rosa: Oona!

Val: Let’s see. Oona’s was 32 steps away and yours was 60. So Oona might be kind of close to your hammock, but who else has a distance that was similar to 60?

Eligh: Psilas! Psilas is 69 steps. 

Val: Yeah, so Rosa and Psilas!

Rosa: We both have 60!

Eligh: It’s just 9 more steps Rosa!

Immy: How far was Xyla? 90?! That’s so many steps! How far were you Leo? 

Leo: 7!

Immy: Was anyone else less than 7? Maybe you’re the closest Leo.

Val: I don’t think anyone is less than 7 yet. I see that Bea was 11 steps which is pretty close to 7. 

Interestingly when Psilas, Immy, and Ember went to add their paths from the fort to their hammock on the map, they counted each drawn dash with the intention of representing each step with a line. There wasn’t space on our paper for them to include all of their steps, and we started to wonder if it might feel good to make a new, more accurate map using a universal symbol for a step. 

On Thursday, Charlie visited our basecamp, and was invited by some friends to use the map of Hammock Valley to find their hammocks. Ember led Charlie on the 105 step journey to her hammock, but strangely enough it only took Charlie 70 steps to get there!

After every friend added themselves to the map, Val created a chart of their estimated footsteps next to the actual footsteps it took them to reach their hammocks. On Friday morning after All School, we held an indoor snack meeting to reflect on what we noticed about our map and our chart.

What do you notice about the map and the chart of numbers?

Noa: I guessed mine was 30, but it was actually 28. 

Xyla: Two steps lower. 

Val: How did you figure out 2 steps lower?

Noa: Kind of like you count down. 

Rosa: I noticed that my name is there, and 6, and 60. My guess was 6, but my real number was 60, and they both have a 6!

Xyla: My guess was 24, but actually 90 was right! It was like 100 further. 

Ember: I guessed 44 of how many steps, but it was actually 105!

Rowan: My guess was 25, and I actually got 60, I should have guessed 60!

Psilas: Leo was the closest to The Big Fort. How I knew that was I tested it when we were playing because for me it was 6 and for Xyla it was 7 because my feet were bigger. 

Val: That reminds me Ember do you remember what happened when Charlie tried to follow the footsteps map to get to your hammock?

Ember: Yeah it was actually like 70 because bigger feet = less steps. And also I left like no gaps in between my feet, but Charlie left gaps. 

Eligh: My guess was 35, but the actual was 55. They both end in 5s!

Grace: I noticed that the hammocks were all different I think.

Val: Oh interesting observation Grace. Is that true? 

Xyla: (noticing Rowan and Rosa are the same) Wait! 60 and 60! 

Rowan: Oh! Okay so Rowan 60 and Rosa 60. Our hammocks are the same steps. 

Ember: My hammock was the furthest one. The biggest number. 

Twilight: I guessed 15, but it was actually 33. 

Ruby: I’m noticing that some people started from the same branch of The Big Fort, but others from different. 

Immy: Hmm let me think. I’m noticing everyone thought of a number, but nobody was right. 

Val: That’s true. Who was the closest to their estimate? 

Ember: Leo and Noa were the same. They are both 2 steps different. 

Val: Oh totally yeah! Leo thought 5, but it was actually 7 which is 2 steps further. Noa thought 30, but it was actually 28 which is 2 steps closer. 

Florence: My guess was 30, but it was actually 75. It wasn’t close at all.

We ended our week on Friday afternoon with the initiation of a large bar graph. Florence and Ruby worked together to add the names of everyone in our group at the base of the graph, and a few friends even had time to color in their bar.

Xyla: Ember’s was definitely the biggest one. I thought mine was the biggest, but Ember’s is actually bigger. How did she even count that high? Hers goes actually off of the paper!

We’re looking forward to seeing how this different way of representing our data will invite even more mathematical observations!